As A Stream's Velocity Decreases, Which Of The Following Sizes Of Sediments Will Be Deposited Last?

Chapter 13 Streams and Floods

13.3 Stream Erosion and Deposition

Every bit we discussed in Chapter 6, flowing water is a very important machinery for erosion, transportation and deposition of sediments. Water period in a stream is primarily related to the stream's gradient, only it is besides controlled by the geometry of the stream channel. Equally shown in Figure xiii.3.1, water flow velocity is decreased by friction forth the stream bed, so information technology is slowest at the bottom and edges and fastest nearly the surface and in the middle. In fact, the velocity just beneath the surface is typically a little higher than right at the surface because of friction between the water and the air. On a curved section of a stream, menstruum is fastest on the outside and slowest on the inside.

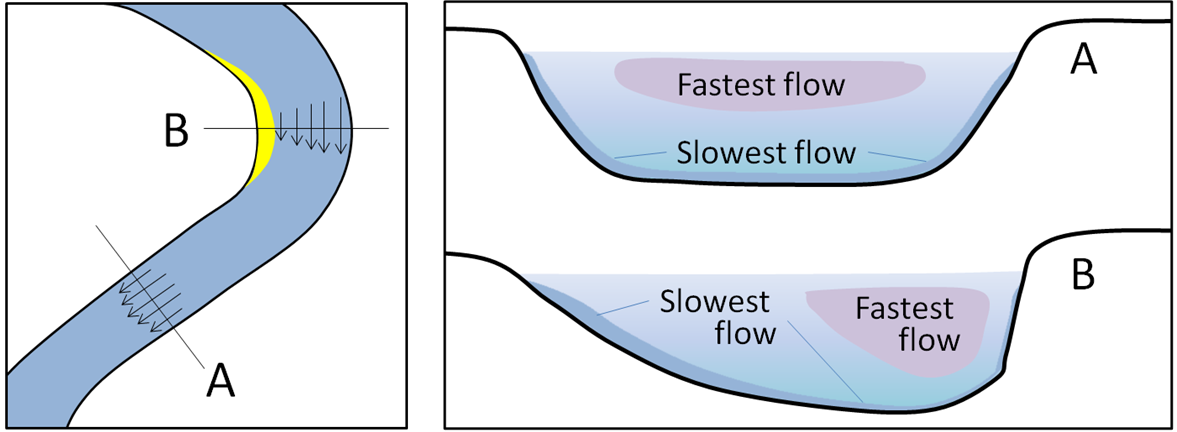
Figure xiii.three.1 The relative velocity of stream menses depending on whether the stream channel is straight or curved (left), and with respect to the h2o depth (right). [Image Description]

Other factors that bear on stream-h2o velocity are the size of sediments on the stream bed—because large particles tend to deadening the menstruum more than than small ones—and the , or book of water passing a signal in a unit of measurement of time (eastward.g., cubic metres (yard³) per second). During a inundation, the water level always rises, so there is more than cross-sectional surface area for the water to menses in, however, as long equally a river remains confined to its channel, the velocity of the water flow too increases.

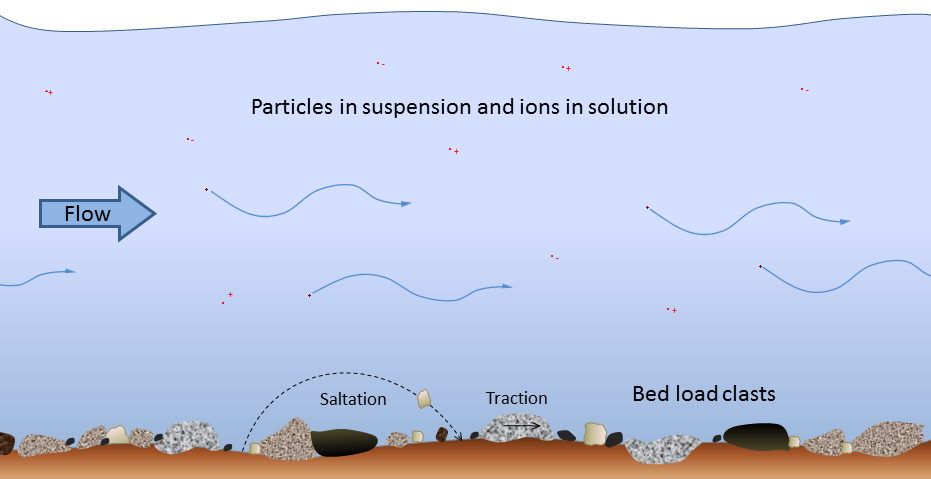
Effigy 13.iii.2 shows the nature of sediment transportation in a stream. Large particles rest on the lesser——and may only be moved during rapid flows under inundation conditions. They can be moved by (billowy) and past (being pushed forth by the force of the menstruum).

Smaller particles may residual on the bottom some of the time, where they can be moved by saltation and traction, but they tin can also exist held in break in the flowing h2o, especially at college velocities. Every bit you know from intuition and from experience, streams that flow fast tend to be turbulent (catamenia paths are chaotic and the h2o surface appears crude) and the water may be muddy, while those that menstruation more than slowly tend to have laminar flow (straight-line flow and a shine water surface) and clear water. Turbulent menstruation is more than effective than laminar catamenia at keeping sediments in interruption.

Stream water also has a dissolved load, which represents (on average) about 15% of the mass of textile transported, and includes ions such every bit calcium (Ca⁺ⁱⁱ) and chloride (Cl⁻) in solution. The solubility of these ions is non affected by period velocity.

Figure thirteen.3.3 Modes of transportation of sediments and dissolved ions (represented past red dots with + and − signs) in a stream.

The faster the water is flowing, the larger the particles that can be kept in suspension and transported within the flowing water. Nonetheless, every bit Swedish geographer Filip Hjulström discovered in the 1940s, the relationship betwixt grain size and the likelihood of a grain being eroded, transported, or deposited is non as simple as ane might imagine (Figure 13.3.3). Consider, for example, a 1 millimetre grain of sand. If information technology is resting on the bottom, it volition remain there until the velocity is loftier enough to erode it, around 20 centimetres per second (cm/s). But once information technology is in suspension, that same 1 mm particle volition remain in suspension as long equally the velocity doesn't drop below ten cm/s. For a x mm gravel grain, the velocity is 105 cm/s to exist eroded from the bed only only lxxx cm/s to remain in suspension.

Figure 13.3.3 The Hjulström-Sundborg diagram showing the relationships between particle size and the trend to be eroded, transported, or deposited at unlike electric current velocities. [Image Description]

On the other hand, a 0.01 mm silt particle only needs a velocity of 0.1 centimetres per second (cm/southward) to remain in suspension, but requires 60 cm/due south to exist eroded. In other words, a tiny silt grain requires a greater velocity to be eroded than a grain of sand that is 100 times larger! For clay-sized particles, the discrepancy is fifty-fifty greater. In a stream, the most easily eroded particles are small-scale sand grains between 0.ii mm and 0.v mm. Annihilation smaller or larger requires a college water velocity to be eroded and entrained in the catamenia. The primary reason for this is that small particles, and specially the tiny grains of clay, have a strong tendency to stick together, and and then are difficult to erode from the stream bed.

It is important to be aware that a stream can both erode and deposit sediments at the same time. At 100 cm/s, for example, silt, sand, and medium gravel will exist eroded from the stream bed and transported in suspension, coarse gravel will be held in suspension, pebbles will exist both transported and deposited, and cobbles and boulders volition remain stationary on the stream bed.

Refer to the Hjulström-Sundborg diagram (Figure 13.3.3) to answer these questions.

A fine sand grain (0.1 millimetres) is resting on the bottom of a stream bed.
1. What stream velocity will it accept to go that sand grain into interruption?
2. Once the particle is in suspension, the velocity starts to drop. At what velocity will it finally come back to rest on the stream bed?
A stream is flowing at 10 centimetres per second (which ways it takes ten seconds to go ane metres, and that's pretty tiresome).
1. What size of particles can exist eroded at x centimetres per second?
2. What is the largest particle that, once already in suspension, will remain in suspension at ten centimetres per second?

Come across Appendix iii for Exercise 13.iii answers.

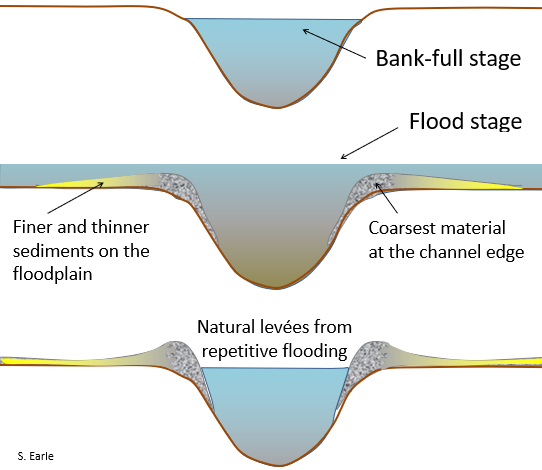
Figure 13.iii.4 The development of natural levées during flooding of a stream. The sediments of the levée get increasingly fine away from the stream aqueduct, and even finer sediments—dirt, silt, and fine sand—are deposited across most of the alluvion plain.

A stream typically reaches its greatest velocity when it is close to flooding over its banks. This is known equally the bank-full stage, as shown in Effigy 13.3.4. As before long every bit the flooding stream overtops its banks and occupies the wide area of its overflowing manifestly, the water has a much larger area to menses through and the velocity drops significantly. At this point, sediment that was existence carried past the loftier-velocity h2o is deposited near the edge of the channel, forming a natural bank or .

Image Descriptions

Figure 13.3.1 image description: When a stream curves, the flow of water is fastest on the outside of the curve and slowest on the inside of the curve. When the stream is straight and a uniform depth, the stream flows fastest in the center nearly the top and slowest along the edges. When the depth is not uniform, the stream flows fastest in the deeper section. [Return to Figure 13.3.1]

Effigy thirteen.iii.3 image description:

Erosion velocity curve: A 0.001 millimetre particle would erode at a catamenia velocity of 500 centimetres per second or greater. As the particle size gets larger, the minimum flow velocity needed to erode the particle decreases, with the everyman menstruation velocity existence 30 centimetres per second to erode a 0.5 millimetre particle. To erode particles larger than 0.v millimetres, the minimum menstruation velocity rises over again.
Settling velocity curve: A 0.01 millimetre particle would be deposited with a flow velocity of 0.1 centimetre per second or less. Equally the flow velocity increases, simply larger and larger particles will be deposited.
Particles between these two curves (either moving likewise slow or existence likewise pocket-size to be eroded or deposited) will be transported in the stream.

[Return to Effigy thirteen.3.3]

Media Attributions